In the previous article, we talked about how to correctly calculate the limits of elementary functions. If we take more complex functions, then expressions with an undefined value will appear in our calculations. They are called uncertainties.

There are the following main types of uncertainties:

1. Division 0 by 0 0 0;
2. Division of one infinity by another ∞ ∞;

0 raised to the zero power 0 0;

3. infinity raised to the zero power ∞ 0.

We have listed all the major uncertainties. Other expressions in different conditions can take on finite or infinite values, therefore, they cannot be considered ambiguities.

Disclosure of uncertainties

Uncertainty can be revealed:

1. By simplifying the form of the function (using abbreviated multiplication formulas, trigonometric formulas, additional multiplication by conjugate expressions and subsequent reduction, etc.);

With wonderful limits;

Using L'Hôpital's rule;

By replacing one infinitesimal expression with its equivalent expression (as a rule, this action is performed using a table of infinitesimal expressions).

All the information presented above can be graphically presented in the form of a table. On the left side, it shows the type of uncertainty, on the right - a suitable method for its disclosure (finding the limit). This table is very useful for calculations related to finding the limits.

Uncertainty	Uncertainty Disclosure Method
1. Division 0 by 0	Transformation and subsequent simplification of the expression. If the expression is of the form sin (k x) k x or k x sin (k x) then you need to use the first remarkable limit. If such a solution does not fit, we use L'Hôpital's rule or the table of equivalent infinitesimal expressions
2. Division of infinity by infinity	Transforming and simplifying an expression or using the L'Hôpital rule
3. Multiplying zero by infinity or finding the difference between two infinities	Conversion to 0 0 or ∞ ∞ followed by L'Hôpital's rule
4. Unit to the power of infinity	Using the second great limit
5. Raising zero or infinity to zero power	Taking the logarithm of the expression using the equality lim x → x 0 ln (f (x)) = ln lim x → x 0 f (x)

Let's take a look at a couple of tasks. These examples are quite simple: in them, the answer is obtained immediately after the substitution of values ​​and there is no ambiguity.

Example 1

Find the limit lim x → 1 x 3 + 3 x - 1 x 5 + 3.

Solution

We perform the substitution of values ​​and get the answer.

lim x → 1 x 3 + 3 x - 1 x 5 + 3 = 1 3 + 3 1 - 1 1 5 + 3 = 3 4 = 3 2

Answer: lim x → 1 x 3 + 3 x - 1 x 5 + 3 = 3 2.

Example 2

Calculate the limit lim x → 0 (x 2 + 2, 5) 1 x 2.

Solution

We have an exponential function, in the base of which you need to substitute x = 0.

(x 2 + 2, 5) x = 0 = 0 2 + 2, 5 = 2, 5

Hence, we can convert the limit to the following expression:

lim x → 0 (x 2 + 2, 5) 1 x 2 = lim x → 0 2, 5 1 x 2

Now let's deal with the exponent - a power function 1 x 2 = x - 2. Let's look at the table of limits for power functions with exponent less than zero and get the following: lim x → 0 + 0 1 x 2 = lim x → 0 + 0 x - 2 = + ∞ and lim x → 0 + 0 1 x 2 = lim x → 0 + 0 x - 2 = + ∞

Thus, we can write that lim x → 0 (x 2 + 2, 5) 1 x 2 = lim x → 0 2, 5 1 x 2 = 2.5 + ∞.

Now we take a table of the limits of exponential functions with bases greater than 0, and we get:

lim x → 0 (x 2 + 2, 5) 1 x 2 = lim x → 0 2, 5 1 x 2 = 2, 5 + ∞ = + ∞

Answer: lim x → 0 (x 2 + 2, 5) 1 x 2 = + ∞.

Example 3

Find the limit lim x → 1 x 2 - 1 x - 1.

Solution

We perform value substitution.

lim x → 1 x 2 - 1 x - 1 = 1 2 - 1 1 - 1 = 0 0

As a result, we got uncertainty. Use the table above to select a solution method. It states that you need to simplify the expression.

lim x → 1 x 2 - 1 x - 1 = 0 0 = lim x → 1 (x - 1) (x + 1) x - 1 = = lim x → 1 (x - 1) (x + 1) (X + 1) x - 1 = lim x → 1 (x + 1) x - 1 = = 1 + 1 1 - 1 = 2 0 = 0

As we can see, simplification has led to the disclosure of uncertainty.

Answer: lim x → 1 x 2 - 1 x - 1 = 0

Example 4

Find the limit lim x → 3 x - 3 12 - x - 6 + x.

Solution

Substitute the value and get a record of the following form.

lim x → 3 x - 3 12 - x - 6 + x = 3 - 3 12 - 3 - 6 + 3 = 0 9 - 9 = 0 0

We have come to the necessity of dividing zero by zero, which is an uncertainty. Let's see the required solution method in the table - this is a simplification and transformation of an expression. Let's perform additional multiplication of the numerator and denominator by the expression 12 - x + 6 + x conjugate to the denominator:

lim x → 3 x - 3 12 - x - 6 + x = 0 0 = lim x → 3 x - 3 12 - x + 6 + x 12 - x - 6 + x 12 - x + 6 + x

The multiplication of the denominator is performed so that later you can use the formula for reduced multiplication (difference of squares) and perform the reduction.

lim x → 3 x - 3 12 - x + 6 + x 12 - x - 6 + x 12 - x + 6 + x = lim x → 3 x - 3 12 - x + 6 + x 12 - x 2 - 6 + x 2 = lim x → 3 (x - 3) 12 - x + 6 + x 12 - x - (6 + x) = = lim x → 3 (x - 3) 12 - x + 6 + x 6 - 2 x = lim x → 3 (x - 3) 12 - x + 6 + x - 2 (x - 3) = = lim x → 3 12 - x + 6 + x - 2 = 12 - 3 + 6 + 3 - 2 = 9 + 9 - 2 = - 9 = - 3

As we can see, as a result of these actions, we managed to get rid of uncertainty.

Answer: lim x → 3 x - 3 12 - x - 6 + x = - 3.

It is important to note that when solving such problems, the multiplication approach is used very often, so we advise you to remember exactly how this is done.

Example 5

Find the limit lim x → 1 x 2 + 2 x - 3 3 x 2 - 5 x + 2.

Solution

We perform substitution.

lim x → 1 x 2 + 2 x - 3 3 x 2 - 5 x + 2 = 1 2 + 2 1 - 3 3 1 2 - 5 1 + 2 = 0 0

As a result, we got uncertainty. The recommended way to solve the problem in this case is to simplify the expression. Since at the value of x equal to one, the numerator and denominator vanish, we can factor them and then cancel them by x - 1, and then the uncertainty will disappear.

We perform the factorization of the numerator:

x 2 + 2 x - 3 = 0 D = 2 2 - 4 1 (- 3) = 16 ⇒ x 1 = - 2 - 16 2 = - 3 x 2 = - 2 + 16 2 = 1 ⇒ x 2 + 2 x - 3 = x + 3 x - 1

Now we do the same with the denominator:

3 x 2 - 5 x + 2 = 0 D = - 5 2 - 4 3 2 = 1 ⇒ x 1 = 5 - 1 2 3 = 2 3 x 2 = 5 + 1 2 3 = 1 ⇒ 3 x 2 - 5 x + 3 = 3 x - 2 3 x - 1

We got the following limit:

lim x → 1 x 2 + 2 x - 3 3 x 2 - 5 x + 2 = 0 0 = lim x → 1 x + 3 x - 1 3 x - 2 3 x - 1 = = lim x → 1 x + 3 3 x - 2 3 = 1 + 3 3 1 - 2 3 = 4

As we can see, during the transformation, we managed to get rid of uncertainty.

Answer: lim x → 1 x 2 + 2 x - 3 3 x 2 - 5 x + 2 = 4.

Next, we need to consider the cases of limits at infinity in exponential expressions. If the exponents of these expressions are greater than 0, then the limit at infinity will also be infinite. In this case, the greatest degree is of primary importance, and the rest can be ignored.

For example, lim x → ∞ (x 4 + 2 x 3 - 6) = lim x → ∞ x 4 = ∞ or lim x → ∞ x 4 + 4 x 3 + 21 x 2 - 11 5 = lim x → ∞ x 4 5 = ∞.

If under the limit sign we have a fraction with exponential expressions in the numerator and denominator, then as x → ∞ we have an uncertainty of the form ∞ ∞. To get rid of this ambiguity, we need to divide the numerator and denominator of the fraction by x m a x (m, n). Let's give an example of solving a similar problem.

Example 6

Find the limit lim x → ∞ x 7 + 2 x 5 - 4 3 x 7 + 12.

Solution

lim x → ∞ x 7 + 2 x 5 - 4 3 x 7 + 12 = ∞ ∞

The powers of the numerator and denominator are 7. We divide them by x 7 and get:

lim x → ∞ x 7 + 2 x 5 - 4 3 x 7 + 12 = lim x → ∞ x 7 + 2 x 5 - 4 x 7 3 x 7 + 12 x 7 = = lim x → ∞ 1 + 2 x 2 - 4 x 7 3 + 12 x 7 = 1 + 2 ∞ 2 - 4 ∞ 7 3 + 12 ∞ 7 = 1 + 0 - 0 3 + 0 = 1 3

Answer: lim x → ∞ x 7 + 2 x 5 - 4 3 x 7 + 12 = 1 3.

Example 7

Calculate the limit lim x → ∞ x 8 + 11 3 x 2 + x + 1.

Solution

lim x → ∞ x 8 + 11 3 x 2 + x + 1 = ∞ ∞

The numerator has degree 8 3 and denominator 2. Let's divide the numerator and denominator by x 8 3:

lim x → ∞ x 8 + 11 3 x 2 + x + 1 = ∞ ∞ = lim x → ∞ x 8 + 11 3 x 8 3 x 2 + x + 1 x 8 3 = = lim x → ∞ 1 + 11 x 8 3 1 x 2 3 + 1 x 5 3 + 1 x 8 3 = 1 + 11 ∞ 3 1 ∞ + 1 ∞ + 1 ∞ = 1 + 0 3 0 + 0 + 0 = 1 0 = ∞

Answer: lim x → ∞ x 8 + 11 3 x 2 + x + 1 = ∞.

Example 8

Calculate the limit lim x → ∞ x 3 + 2 x 2 - 1 x 10 + 56 x 7 + 12 3.

Solution

lim x → ∞ x 3 + 2 x 2 - 1 x 10 + 56 x 7 + 12 3 = ∞ ∞

We have a numerator to the power of 3 and a denominator to the power of 10 3. So we need to divide the numerator and denominator by x 10 3:

lim x → ∞ x 3 + 2 x 2 - 1 x 10 + 56 x 7 + 12 3 = ∞ ∞ = lim x → ∞ x 3 + 2 x 2 - 1 x 10 3 x 10 + 56 x 7 + 12 3 x 10 3 = = lim x → ∞ 1 x 1 3 + 2 x 4 3 - 1 x 10 3 1 + 56 x 3 + 12 x 10 3 = 1 ∞ + 2 ∞ - 1 ∞ 1 + 56 ∞ + 12 ∞ 3 = 0 + 0 - 0 1 + 0 + 0 3 = 0

Answer: lim x → ∞ x 3 + 2 x 2 - 1 x 10 + 56 x 7 + 12 3 = 0.

conclusions

In the case of the relationship limit, there are three main options:

If the degree of the numerator is equal to the degree of the denominator, then the limit will be equal to the ratio of the coefficients at the highest degrees.

If the degree of the numerator is greater than the degree of the denominator, then the limit will be equal to infinity.

If the degree of the numerator is less than the degree of the denominator, then the limit will be zero.

We will discuss other methods of disclosing uncertainties in separate articles.

If you notice an error in the text, please select it and press Ctrl + Enter

The derivative of the function does not fall very far, and in the case of L'Hôpital's rules, it falls exactly in the same direction as the original function. This circumstance helps in disclosing uncertainties of the form 0/0 or ∞ / ∞ and some other uncertainties that arise when calculating limit the ratio of two infinitesimal or infinitely large functions. The calculation is greatly simplified with the help of this rule (in fact, two rules and notes to them):

As the formula above shows, when calculating the limit of the ratio of two infinitesimal or infinitely large functions, the limit of the ratio of two functions can be replaced by the limit of their ratio derivatives and thus get a certain result.

Let's move on to more precise formulations of L'Hôpital's rules.

L'Hôpital's rule for the case of the limit of two infinitesimal quantities... Let the functions f(x) and g(x a... And at the very point a a derivative of a function g(x) is not equal to zero ( g"(x a are equal to each other and equal to zero:

.

L'Hôpital's rule for the case of the limit of two infinitely large quantities... Let the functions f(x) and g(x) have derivatives (that is, differentiable) in some neighborhood of the point a... And at the very point a they may or may not have derivatives. Moreover, in the vicinity of the point a derivative of a function g(x) is not equal to zero ( g"(x) ≠ 0) and the limits of these functions as x tends to the value of the function at the point a are equal to each other and equal to infinity:

.

Then the limit of the ratio of these functions is equal to the limit of the ratio of their derivatives:

In other words, for uncertainties of the form 0/0 or ∞ / ∞, the limit of the ratio of two functions is equal to the limit of the ratio of their derivatives, if the latter exists (finite, that is, equal to a certain number, or infinite, that is, equal to infinity).

Remarks.

1. L'Hôpital's rules are also applicable when the functions f(x) and g(x) are not defined for x = a.

2. If, when calculating the limit of the ratio of derivatives of functions f(x) and g(x) again we arrive at an uncertainty of the form 0/0 or ∞ / ∞, then L'Hôpital's rules should be applied multiple times (at least twice).

3. L'Hôpital's rules are also applicable when the argument of the functions (x) tends not to a finite number a, and to infinity ( x → ∞).

Uncertainties of other types can also be reduced to uncertainties of the 0/0 and ∞ / ∞ types.

Disclosure of uncertainties of the types "zero divided by zero" and "infinity divided by infinity"

Example 1.

x= 2 results in an uncertainty of the form 0/0. Therefore, the derivative of each function and we obtain

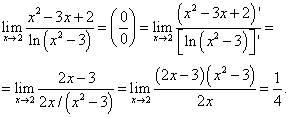
The derivative of the polynomial was calculated in the numerator, and in the denominator - derivative of a complex logarithmic function... Before the last equal sign, the usual limit, substituting two instead of x.

Example 2. Calculate the limit of the ratio of two functions using L'Hôpital's rule:

Solution. Substitution of a value in a given function x

Example 3. Calculate the limit of the ratio of two functions using L'Hôpital's rule:

Solution. Substitution of a value in a given function x= 0 results in an uncertainty of the form 0/0. Therefore, we calculate the derivatives of the functions in the numerator and denominator and get:

Example 4. Calculate

Solution. Substitution of the x value equal to plus infinity into the given function leads to an uncertainty of the form ∞ / ∞. Therefore, we apply L'Hôpital's rule:

Comment. Let us turn to examples in which L'Hôpital's rule has to be applied twice, that is, to come to the limit of the ratios of the second derivatives, since the limit of the ratio of the first derivatives is an uncertainty of the form 0/0 or ∞ / ∞.

Disclosure of uncertainties of the form "zero times infinity"

Example 12. Calculate

.

Solution. We get

This example uses trigonometric identity.

Disclosure of uncertainties of the types "zero to the power of zero", "infinity to the power of zero" and "one to the power of infinity"

Uncertainties of the form, or are usually reduced to the form 0/0 or ∞ / ∞ using the logarithm of a function of the form

To calculate the limit of an expression, one should use the logarithmic identity, a special case of which is the property of the logarithm .

Using the logarithmic identity and the continuity property of the function (to go beyond the limit sign), the limit should be calculated as follows:

Separately, you should find the limit of expression in the exponent and build e to the degree found.

Example 13.

Solution. We get

.

.

Example 14. Calculate using L'Hôpital's rule

Solution. We get

We calculate the limit of expression in the exponent

.

.

Example 15. Calculate using L'Hôpital's rule

Methods for solving the limits. Uncertainties.
The growth order of the function. Replacement method

Example 4

Find the limit

This is a simpler example for a do-it-yourself solution. In the proposed example, there is again uncertainty (of a higher order of growth than the root).

If "x" tends to "minus infinity"

The ghost of "minus infinity" has been in this article for a long time. Consider the limits with polynomials in which. The principles and methods of solution will be exactly the same as in the first part of the lesson, with the exception of a number of nuances.

Consider 4 chips that will be required to solve practical tasks:

1) Calculate the limit

The value of the limit depends only on the term, since it has the highest growth order. If, then infinitely large modulo negative number to the EVEN power, in this case - in the fourth, equal to "plus infinity":. Constant ("two") positive, therefore:

2) Calculate the limit

Here again the senior degree even, therefore: . But in front of the "minus" ( negative constant -1), therefore:

3) Calculate the limit

The limit value depends only on. As you remember from school, the "minus" "jumps out" from under the odd degree, so infinitely large modulo negative number to odd power equals "minus infinity", in this case:.
Constant ("four") positive, means:

4) Calculate the limit

The first guy in the village has again odd degree, moreover, in the bosom negative constant, which means: Thus:
.

Example 5

Find the limit

Using the above points, we come to the conclusion that there is uncertainty. The numerator and denominator are of the same order of growth, which means that in the limit you get a finite number. Let's find out the answer, discarding all the fry:

The solution is trivial:

Example 6

Find the limit

This is an example for a do-it-yourself solution. Complete solution and answer at the end of the tutorial.

And now, perhaps the most subtle of the cases:

Example 7

Find the limit

Considering the leading terms, we come to the conclusion that there is uncertainty here. The numerator is of a higher order of growth than the denominator, so you can immediately say that the limit is equal to infinity. But which infinity, plus or minus? The technique is the same - in the numerator and denominator, we will get rid of the little things:

We decide:

Divide the numerator and denominator by

Example 15

Find the limit

This is an example for a do-it-yourself solution. A rough example of finishing at the end of the lesson.

A couple more interesting examples on variable replacement:

Example 16

Find the limit

Substituting one into the limit results in uncertainty. Variable replacement is already obvious, but first we transform the tangent using the formula. Indeed, why do we need a tangent?

Note that, therefore. If not entirely clear, look at the sine values ​​in trigonometric table... Thus, we immediately get rid of the multiplier, in addition, we get the more familiar 0: 0 uncertainty. It would also be nice if our limit tends to zero.

Let's replace:

If, then

Under the cosine we have "x", which also needs to be expressed through "te".
From the replacement we express:.

We complete the solution:

(1) We perform the substitution

(2) Expand the brackets under the cosine.

(4) To organize first wonderful limit, artificially multiply the numerator by and the reciprocal.

Assignment for an independent solution:

Example 17

Find the limit

Complete solution and answer at the end of the tutorial.

These were not difficult tasks in their class, in practice everything can be worse, and, in addition to reduction formulas, you have to use a variety of trigonometric formulas, as well as other tricks. In the article Difficult Limits, I took apart a couple of real examples =)

On the eve of the holiday, let us finally clarify the situation with one more widespread uncertainty:

Elimination of uncertainty "one to the degree of infinity"

This uncertainty is "served" second wonderful limit, and in the second part of that lesson, we considered in great detail the standard examples of solutions that in most cases are encountered in practice. Now the picture with the exhibitors will be completed, in addition, the final tasks of the lesson will be devoted to the limits-"trick", in which it SEEMS that it is necessary to apply the 2nd wonderful limit, although this is not at all the case.

The disadvantage of the two working formulas of the 2nd remarkable limit is that the argument must tend to "plus infinity" or to zero. But what if the argument tends to a different number?

A universal formula comes to the rescue (which is actually a consequence of the second remarkable limit):

Uncertainty can be eliminated by the formula:

Somewhere like already explained what square brackets mean. Nothing special, brackets are like brackets. They are usually used to make a mathematical notation clearer.

Let's highlight the essential points of the formula:

1) It is only about uncertainty and no other.

2) The "x" argument may tend to arbitrary value(and not only to zero or), in particular, to "minus infinity" or to any a finite number.

Using this formula, you can solve all the examples of the lesson. Wonderful limits which belong to the 2nd remarkable limit. For example, let's calculate the limit:

In this case , and by the formula :

True, I do not advise you to do so; in the tradition, you should still use the "usual" design of the solution, if it can be applied. but using a formula is very convenient to check"Classic" examples at the 2nd remarkable limit.

This uncertainty is "served" second wonderful limit, and in the second part of that lesson, we considered in great detail the standard examples of solutions that in most cases are encountered in practice. Now the picture with the exhibitors will be completed, in addition, the final tasks of the lesson will be devoted to the limits-"trick", in which it SEEMS that it is necessary to apply the 2nd wonderful limit, although this is not at all the case.

The disadvantage of the two working formulas of the 2nd remarkable limit is that the argument must tend to "plus infinity" or to zero. But what if the argument tends to a different number?

A universal formula comes to the rescue (which is actually a consequence of the second remarkable limit):

Uncertainty can be eliminated by the formula:

Somewhere like already explained what square brackets mean. Nothing special, brackets are like brackets. They are usually used to make a mathematical notation clearer.

Let's highlight the essential points of the formula:

1) It is only about certainty and no other.

2) The "x" argument may tend to arbitrary value(and not only to zero or), in particular, to "minus infinity" or to any a finite number.

Using this formula, you can solve all the examples of the lesson. Wonderful limits which belong to the 2nd remarkable limit. For example, let's calculate the limit:

In this case , and by the formula :

True, I do not advise you to do so; in the tradition, you should still use the "usual" design of the solution, if it can be applied. but using a formula is very convenient to check"Classic" examples at the 2nd remarkable limit.

All this is good, right, but now there are more interesting shots in the frame:

Example 18

Calculate Limit

At the first step, I will not tire of repeating, we substitute the value "x" in the expression under the limit sign. What if there is no uncertainty at all? It happens! But not at this time. Substituting the "three", we come to the conclusion that here the uncertainty

We use the formula

In order not to drag the letter "e" along and not to be small, the indicator it is more convenient to calculate separately:

In this case:

Thus:

From the point of view of computational techniques, everything is routine: first we bring the first term to a common denominator, then we take out the constants and carry out reductions, getting rid of the 0: 0 uncertainty.

As a result:

The promised gift with the difference in logarithms and uncertainty:

Example 19

Calculate Limit

First the complete solution, then the comments:

(1) - (2) At the first two steps, we use the formulas ... Have complex derivatives we “break up” the logarithms, but here, on the contrary, they need to be “collected”.

(3) Move the limit icon under the logarithm. This can be done because the given logarithm continuous to "minus infinity". In addition, the limit refers to the "stuffing" of the logarithm.

(4) - (5) The standard technique discussed in the basic lesson about wonderful limits, we transform the uncertainty to the form.

(6) We use the formula .

(7) The exponential and logarithmic functions are mutually inverse functions, therefore both "e" and the logarithm can be removed. Indeed, according to the property of the logarithm:. We add the minus before the fraction to the denominator:

(8) No comment =)

The considered type of limit is not so rare, I have found examples of 30-40.

Example 20

Calculate Limit

This is an example for a do-it-yourself solution. In addition to using the formula, the limit can be represented as and by replacing, reduce the solution to the case .

In conclusion, consider the limits of the "fake".

Let's get back to the uncertainty. This uncertainty not always can be reduced to uncertainty and use the 2nd remarkable limit or the formula-consequence. The conversion is feasible if the numerator and denominator of the base of the degree - equivalent infinitely large functions... For example: .

Let's digress from the indicator and calculate the base limit:

In the limit received unit, so the numerator and denominator not just of the same order of growth, but also equivalent... At the lesson Wonderful limits. Examples of solutions we reduced this example to uncertainty without any problems and received an answer.

You can think of a lot of similar limits:
etc.

The fractions of these examples are united by the above feature:. In other cases, with uncertainty 2nd wonderful limit does not apply.

Example 21

Find the limits

No matter how hard you try, uncertainty cannot be transformed into uncertainty.

Here the numerators and denominators of the bases same order of growth, but not equivalents: .

Thus, the second remarkable limit and, moreover, the formula, CANNOT APPLY.

! Note: not to be confused with Example # 18, where the numerator and denominator of the base are not equivalent. There is ready-made uncertainty, but here we are talking about uncertainty.

The method for solving the “fake” limits is simple and the sign Ohm: you need a numerator and a denominator foundations divided by "x" in the highest degree (regardless of the indicator):

If the numerator and denominator of the base are of different order of growth, then the solution is exactly the same:

Example 22

Find the limits

These are short examples for self-study

Sometimes there may be no uncertainty at all:

Such tricks are especially loved by the compilers of Kuznetsov's collection. That is why it is very important to ALWAYS substitute "x" in the expression under the limit sign in the first step!

Example 2

The highest degree of the numerator: 2; the highest degree of the denominator: 3.
:

Example 4

Divide the numerator and denominator by :


Note : the very last action multiplied the numerator and denominator by to get rid of the irrationality in the denominator.

Example 6

Divide the numerator and denominator by :

Example 8

Divide the numerator and denominator by :

Note : term going to zero is slower than , therefore is the "main" zero of the denominator. .

Example 22


Note : infinitesimal function tends to zero more slowly than , therefore, the “larger” zero of the denominator plays a decisive role:

This article, "The Second Remarkable Limit," is devoted to disclosing within the following uncertainties:

$ \ bigg [\ frac (\ infty) (\ infty) \ bigg] ^ \ infty $ and $ ^ \ infty $.

Also, such uncertainties can be disclosed using the logarithm of the exponential function, but this is a different solution method, which will be covered in another article.

Formula and Consequences

Formula the second remarkable limit is written as follows: $$ \ lim_ (x \ to \ infty) \ bigg (1+ \ frac (1) (x) \ bigg) ^ x = e, \ text (where) e \ approx 2.718 $$

The formula implies consequences, which are very convenient to use for solving examples with limits: $$ \ lim_ (x \ to \ infty) \ bigg (1 + \ frac (k) (x) \ bigg) ^ x = e ^ k, \ text (where) k \ in \ mathbb (R) $$ $$ \ lim_ (x \ to \ infty) \ bigg (1 + \ frac (1) (f (x)) \ bigg) ^ (f (x)) = e $ $ $$ \ lim_ (x \ to 0) \ bigg (1 + x \ bigg) ^ \ frac (1) (x) = e $$

It is worth noting that the second remarkable limit can be applied not always to the exponential function, but only in cases where the base tends to unity. To do this, first, the base limit is calculated in the mind, and then conclusions are drawn. All of this will be covered in the sample solutions.

Examples of solutions

Let's consider examples of solutions using the direct formula and its consequences. We will also analyze the cases in which the formula is not needed. It is enough to write down only the ready-made answer.

Example 1

Find the limit $ \ lim_ (x \ to \ infty) \ bigg (\ frac (x + 4) (x + 3) \ bigg) ^ (x + 3) $

Solution

Let's substitute infinity in the limit and look at the uncertainty: $$ \ lim_ (x \ to \ infty) \ bigg (\ frac (x + 4) (x + 3) \ bigg) ^ (x + 3) = \ bigg (\ frac (\ infty) (\ infty) \ bigg) ^ \ infty $$ Find the limit of the base: $$ \ lim_ (x \ to \ infty) \ frac (x + 4) (x + 3) = \ lim_ (x \ to \ infty) \ frac (x (1+ \ frac (4) ( x))) (x (1+ \ frac (3) (x))) = 1 $$ Got the foundation equal to one, which means it is already possible to apply the second remarkable limit. To do this, we fit the base of the function to the formula by subtracting and adding one: $$ \ lim_ (x \ to \ infty) \ bigg (1 + \ frac (x + 4) (x + 3) - 1 \ bigg) ^ (x + 3) = \ lim_ (x \ to \ infty) \ bigg (1 + \ frac (1) (x + 3) \ bigg) ^ (x + 3) = $$ We look at the second consequence and write down the answer: $$ \ lim_ (x \ to \ infty) \ bigg (1 + \ frac (1) (x + 3) \ bigg) ^ (x + 3) = e $$ If you can't solve your problem, then send it to us. We will provide a detailed solution. You will be able to familiarize yourself with the course of the calculation and get information. This will help you get credit from your teacher in a timely manner!

Answer

$$ \ lim_ (x \ to \ infty) \ bigg (1 + \ frac (1) (x + 3) \ bigg) ^ (x + 3) = e $$

Example 4

Solve limit $ \ lim_ (x \ to \ infty) \ bigg (\ frac (3x ^ 2 + 4) (3x ^ 2-2) \ bigg) ^ (3x) $

Solution

We find the limit of the base and see that $ \ lim_ (x \ to \ infty) \ frac (3x ^ 2 + 4) (3x ^ 2-2) = 1 $, so the second wonderful limit can be applied. Standardly, according to the plan, we add and subtract one from the base of the degree: $$ \ lim_ (x \ to \ infty) \ bigg (1+ \ frac (3x ^ 2 + 4) (3x ^ 2-2) -1 \ bigg) ^ (3x) = \ lim_ (x \ to \ infty ) \ bigg (1+ \ frac (6) (3x ^ 2-2) \ bigg) ^ (3x) = $$ We fit the fraction to the formula of the 2nd remark. limit: $$ = \ lim_ (x \ to \ infty) \ bigg (1+ \ frac (1) (\ frac (3x ^ 2-2) (6)) \ bigg) ^ (3x) = $$ Now let's adjust the degree. The power must be a fraction equal to the denominator of the base $ \ frac (3x ^ 2-2) (6) $. To do this, multiply and divide the degree by it, and continue to solve: $$ = \ lim_ (x \ to \ infty) \ bigg (1+ \ frac (1) (\ frac (3x ^ 2-2) (6)) \ bigg) ^ (\ frac (3x ^ 2-2) (6) \ cdot \ frac (6) (3x ^ 2-2) \ cdot 3x) = \ lim_ (x \ to \ infty) e ^ (\ frac (18x) (3x ^ 2-2)) = $$ The limit located in degree at $ e $ is: $ \ lim_ (x \ to \ infty) \ frac (18x) (3x ^ 2-2) = 0 $. Therefore, continuing the solution, we have:

Answer

$$ \ lim_ (x \ to \ infty) \ bigg (\ frac (3x ^ 2 + 4) (3x ^ 2-2) \ bigg) ^ (3x) = 1 $$

Let us examine the cases when the problem is similar to the second remarkable limit, but it can be solved without it.

In the article: "The second remarkable limit: examples of solutions" was analyzed the formula, its consequences and given the frequent types of problems on this topic.